Quantum Error Correction and the Floquet Codes
Julien Bréhier
University of Bonn March 14, 2025

Journey Through Quantum Error Correction
- Introduction
- The Toric Code
- The Toric Honeycomb Floquet Code (THFC)
- Cutting Slices
- Transversal Operators
- Lattice Surgery (LS)
- Prospects
Important notions
-
Stabilizer codes
-
Distance of a code
-
'Good codes'
-
Logical operators
-
Minimum Weight Perfect Matching
-
Ancilla measurements
-
Error Correcting
-
Fault tolerance
-
Transversal operators
-
Lattice Surgery
Stabilizer codes
Stabilizer codes
Stabilizer codes
Stabilizer codes
Stabilizer codes
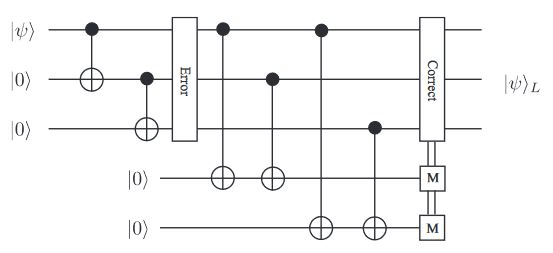
The 3-Qubit Code
The 3-Qubit Code
https://arxiv.org/pdf/0905.2794
The 3-Qubit Code

https://arxiv.org/pdf/0905.2794
The 3-Qubit Code

https://arxiv.org/pdf/0905.2794
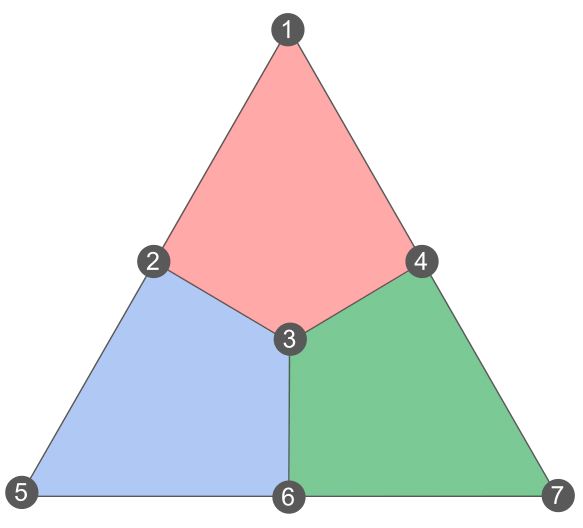
The Steane Code
https://arthurpesah.me/blog/2023-03-16-stabilizer-formalism-2/
The Steane Code

https://arthurpesah.me/blog/2023-03-16-stabilizer-formalism-2/
The Steane Code

https://arthurpesah.me/blog/2023-03-16-stabilizer-formalism-2/
The Search for Good Codes
The Search for Good Codes
You might remember :
The Search for Good Codes
You might remember :
Journey Through Quantum Error Correction
- Introduction
- The Toric Code
- The Toric Honeycomb Floquet Code (THFC)
- Cutting Slices
- Transversal Operators
- Lattice Surgery (LS)
- Prospects
Hamiltonian
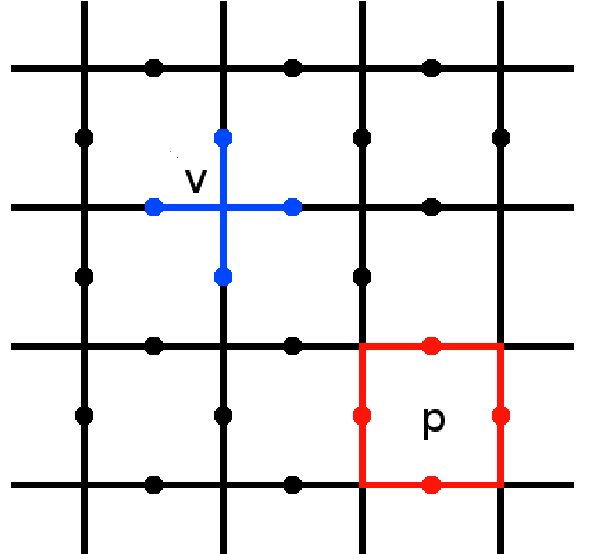
Logical Operators
https://errorcorrectionzoo.org/c/toric
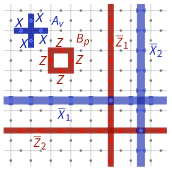
Minimum Weight Perfect Matching
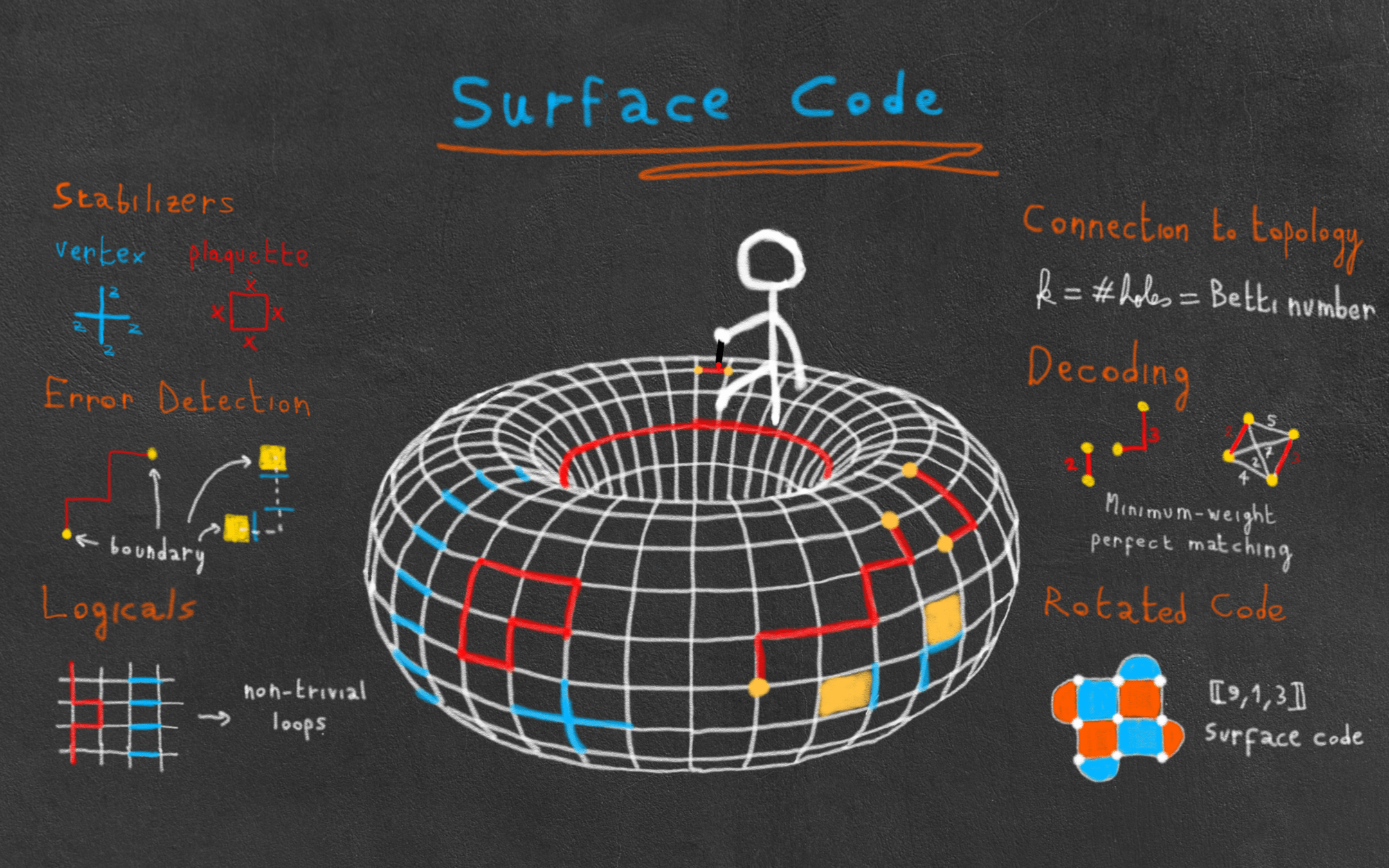
https://arthurpesah.me/blog/2023-05-13-surface-code/
Journey Through Quantum Error Correction
- Introduction
- The Toric Code
- The Toric Honeycomb Floquet Code (THFC)
- Cutting Slices
- Transversal Operators
- Lattice Surgery (LS)
- Prospects

Measurement Method Through Ancilla

Other Measurements

Kitaev Spin Model
THC Yields No Qubit

Floquet-ness

Toric Code ?

Two Protected Operators
Journey Through Quantum Error Correction
- Introduction
- The Toric Code
- The Toric Honeycomb Floquet Code (THFC)
- Cutting Slices
- Transversal Operators
- Lattice Surgery (LS)
- Prospects
Setting boundaries

https://quantumcomputing.stackexchange.com/questions/4395/how-is-computation-done-in-a-2d-surface-code-array



The Surface Code

The Planar Honeycomb Floquet Code (PHFC)
Careful with the edges

Notion of threshold


Journey Through Quantum Error Correction
- Introduction
- The Toric Code
- The Toric Honeycomb Floquet Code (THFC)
- Cutting Slices
- Transversal Operators
- Lattice Surgery (LS)
- Prospects
Idea of Fault Tolerance
The Steane Code
The Steane Code
Surface Code Example

Surface Code Example

PHFC Example
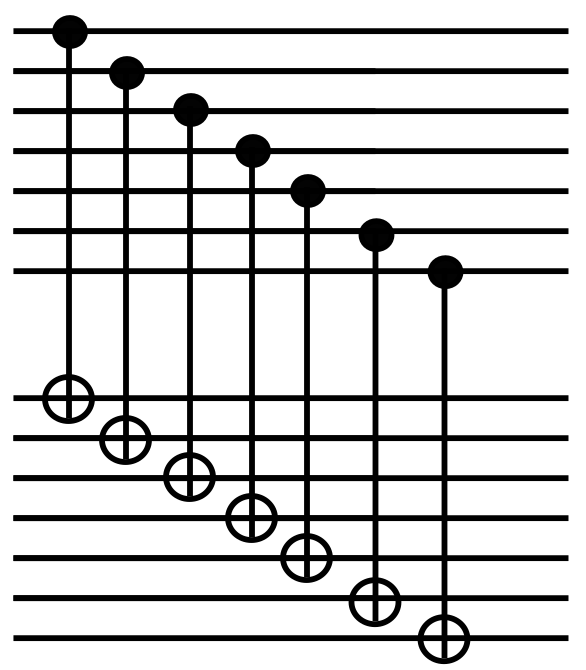
https://quantumcomputing.stackexchange.com/questions/24269/what-is-formally-a-transversal-operator
PHFC Example

https://quantumcomputing.stackexchange.com/questions/24269/what-is-formally-a-transversal-operator

PHFC Example
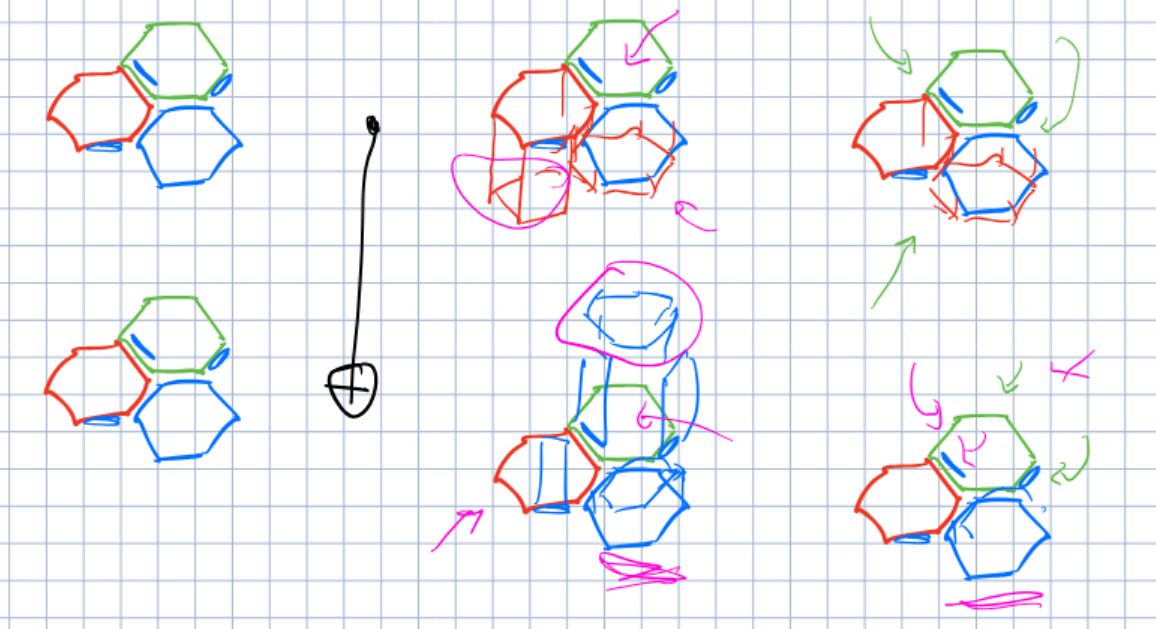
Journey Through Quantum Error Correction
- Introduction
- The Toric Code
- The Toric Honeycomb Floquet Code (THFC)
- Cutting Slices
- Transversal Operators
- Lattice Surgery (LS)
- Prospects
What is it ?

CNot gate
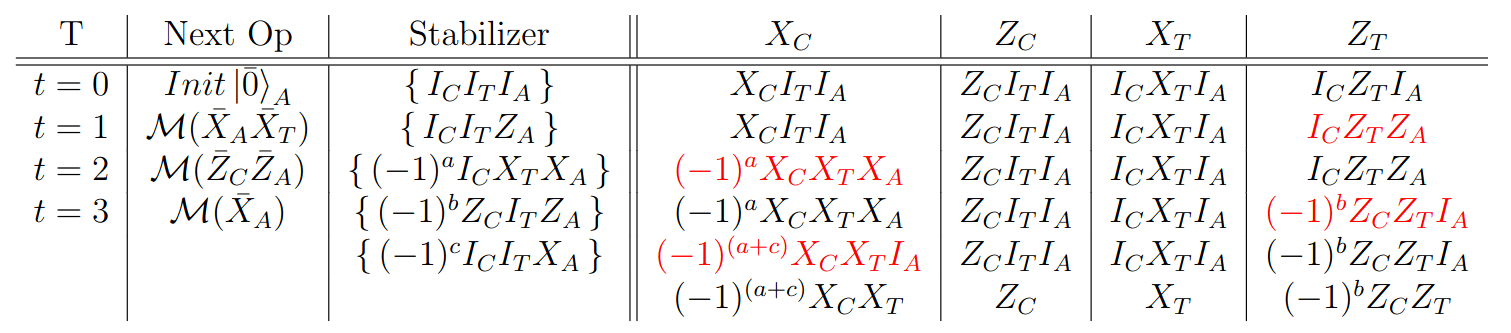
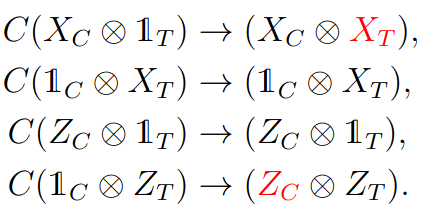
CNot gate
Space time diagram

Journey Through Quantum Error Correction
- Introduction
- The Toric Code
- The Toric Honeycomb Floquet Code (THFC)
- Cutting Slices
- Transversal Operators
- Lattice Surgery (LS)
- Prospects
Important notions
-
Stabilizer codes
-
Distance of a code
-
'Good codes'
-
Logical operators
-
Minimum Weight Perfect Matching
-
Ancilla measurements
-
Error Correcting
-
Fault tolerance
-
Transversal operators
-
Lattice Surgery
Future ideas
- Publish the results comparing the two techniques
- Compare different implementations of the planar codes
- Test experimentally
Quantum Error Correction and the Floquet Codes
By Julien Bréhier
Quantum Error Correction and the Floquet Codes
- 1



