Introducción al control óptimo
IE3041 - Sistemas de Control 2
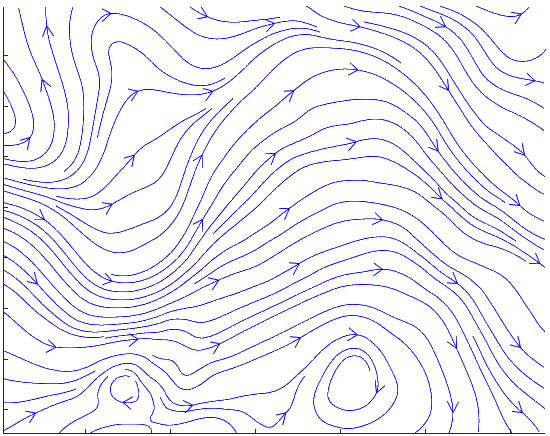
¿Dónde estamos por el momento?
condición inicial \(\mathbf{x}_0\)
"meta" \(\mathbf{x}_{ss}\)
¿Dónde estamos por el momento?
condición inicial \(\mathbf{x}_0\)
"meta" \(\mathbf{x}_{ss}\)
control LTI local
\(\mathbf{x}_0\) debe de estar dentro de la región de atracción para que funcione
¿Dónde estamos por el momento?
condición inicial \(\mathbf{x}_0\)
"meta" \(\mathbf{x}_{ss}\)
control LTI local
\(\mathbf{x}_0\) debe de estar dentro de la región de atracción para que funcione
¿Dónde estamos por el momento?
condición inicial \(\mathbf{x}_0\)
"meta" \(\mathbf{x}_{ss}\)
control LTI local
\(\mathbf{x}_0\) debe de estar dentro de la región de atracción para que funcione
¿Dónde estamos por el momento?
condición inicial \(\mathbf{x}_0\)
"meta" \(\mathbf{x}_{ss}\)
control LTI de alta ganancia?
¿Dónde estamos por el momento?
condición inicial \(\mathbf{x}_0\)
"meta" \(\mathbf{x}_{ss}\)
control LTI de alta ganancia?
\(\Rightarrow\) puede requerir demasiado esfuerzo de control
¿Dónde estamos por el momento?
condición inicial \(\mathbf{x}_0\)
"meta" \(\mathbf{x}_{ss}\)
estrategias como gain-scheduling?
¿Dónde estamos por el momento?
condición inicial \(\mathbf{x}_0\)
"meta" \(\mathbf{x}_{ss}\)
estrategias como gain-scheduling?
\(\Rightarrow\) asumen una visión demasiado LTI de la realidad (?)
¿Dónde estamos por el momento?
el problema con estas estrategias es que son sólo "decentes" a pesar de requerir una gran cantidad de manipulaciones matemáticas
¿Dónde estamos por el momento?
el problema con estas estrategias es que son sólo "decentes" a pesar de requerir una gran cantidad de manipulaciones matemáticas
esta visión local del mundo impide que el controlador explote la dinámica del sistema
¿Dónde estamos por el momento?
el problema con estas estrategias es que son sólo "decentes" a pesar de requerir una gran cantidad de manipulaciones matemáticas
esta visión local del mundo impide que el controlador explote la dinámica del sistema
¿Qué estamos buscando?
¿Dónde estamos por el momento?
condición inicial \(\mathbf{x}_0\)
"meta" \(\mathbf{x}_{ss}\)
¿Dónde estamos por el momento?
condición inicial \(\mathbf{x}_0\)
"meta" \(\mathbf{x}_{ss}\)
se aprovecha el flujo para una estrategia más eficiente
¿Dónde estamos por el momento?
condición inicial \(\mathbf{x}_0\)
"meta" \(\mathbf{x}_{ss}\)
se aprovecha el flujo para una estrategia más eficiente
óptima
\(\Rightarrow\) control óptimo
¿Dónde estamos por el momento?
Control óptimo
problema de control formulado como búsqueda
similar a los casos de optimización "simples", el control óptimo busca resolver un problema específico de optimización pero más complejo | abstracto
Control óptimo
problema de control formulado como búsqueda
la solución es una trayectoria específica, por lo que el problema también se conoce como optimización de trayectorias
similar a los casos de optimización "simples", el control óptimo busca resolver un problema específico de optimización pero más complejo | abstracto
Control óptimo
problema de control formulado como búsqueda
\begin{aligned}
\mathbf{u}^\star(t) = & \displaystyle\arg\min_{\mathbf{u(t)}} \displaystyle\int_{t_0}^{t_f} \mathcal{L}\left(\mathbf{x}(\tau), \mathbf{u}(\tau) \right)d\tau + \varphi\left(\mathbf{x}_0,\mathbf{x}_f\right) \\
& \textrm{s.t.} \quad \dot{\mathbf{x}}=\mathbf{f}\left(\mathbf{x}, \mathbf{u}\right) \\
\end{aligned}
El problema de control óptimo
\begin{aligned}
\mathbf{u}^\star(t) = & \displaystyle\arg\min_{\mathbf{u(t)}} \displaystyle\int_{t_0}^{t_f} \mathcal{L}\left(\mathbf{x}(\tau), \mathbf{u}(\tau) \right)d\tau + \varphi\left(\mathbf{x}_0,\mathbf{x}_f\right) \\
& \textrm{s.t.} \quad \dot{\mathbf{x}}=\mathbf{f}\left(\mathbf{x}, \mathbf{u}\right) \\
\end{aligned}
\(=J\left(\mathbf{u}(t)\right)\) funcional de costo
El problema de control óptimo
\begin{aligned}
\mathbf{u}^\star(t) = & \displaystyle\arg\min_{\mathbf{u(t)}} \displaystyle\int_{t_0}^{t_f} \mathcal{L}\left(\mathbf{x}(\tau), \mathbf{u}(\tau) \right)d\tau + \varphi\left(\mathbf{x}_0,\mathbf{x}_f\right) \\
& \textrm{s.t.} \quad \dot{\mathbf{x}}=\mathbf{f}\left(\mathbf{x}, \mathbf{u}\right) \\
\end{aligned}
horizonte finito
El problema de control óptimo
\begin{aligned}
\mathbf{u}^\star(t) = & \displaystyle\arg\min_{\mathbf{u(t)}} \displaystyle\int_{t_0}^{t_f} \mathcal{L}\left(\mathbf{x}(\tau), \mathbf{u}(\tau) \right)d\tau + \varphi\left(\mathbf{x}_0,\mathbf{x}_f\right) \\
& \textrm{s.t.} \quad \dot{\mathbf{x}}=\mathbf{f}\left(\mathbf{x}, \mathbf{u}\right) \\
\end{aligned}
\mathbf{x_0} \\
t_0
\mathbf{x_f} \\
t_f
El problema de control óptimo
\begin{aligned}
\mathbf{u}^\star(t) = & \displaystyle\arg\min_{\mathbf{u(t)}} \displaystyle\int_{t_0}^{t_f} \mathcal{L}\left(\mathbf{x}(\tau), \mathbf{u}(\tau) \right)d\tau + \varphi\left(\mathbf{x}_0,\mathbf{x}_f\right) \\
& \textrm{s.t.} \quad \dot{\mathbf{x}}=\mathbf{f}\left(\mathbf{x}, \mathbf{u}\right) \\
\end{aligned}
\mathbf{x_0} \\
t_0
\mathbf{x_f} \\
t_f
costo de parqueo o de frontera
El problema de control óptimo
\begin{aligned}
\mathbf{u}^\star(t) = & \displaystyle\arg\min_{\mathbf{u(t)}} \displaystyle\int_{t_0}^{t_f} \mathcal{L}\left(\mathbf{x}(\tau), \mathbf{u}(\tau) \right)d\tau + \varphi\left(\mathbf{x}_0,\mathbf{x}_f\right) \\
& \textrm{s.t.} \quad \dot{\mathbf{x}}=\mathbf{f}\left(\mathbf{x}, \mathbf{u}\right) \\
\end{aligned}
\mathbf{x_0} \\
t_0
\mathbf{x_f} \\
t_f
costo de parqueo o de frontera
costo de trayectoria
El problema de control óptimo
\begin{aligned}
\mathbf{u}^\star(t) = & \displaystyle\arg\min_{\mathbf{u(t)}} \displaystyle\int_{t_0}^{t_f} \mathcal{L}\left(\mathbf{x}(\tau), \mathbf{u}(\tau) \right)d\tau + \varphi\left(\mathbf{x}_0,\mathbf{x}_f\right) \\
& \textrm{s.t.} \quad \dot{\mathbf{x}}=\mathbf{f}\left(\mathbf{x}, \mathbf{u}\right) \\
\end{aligned}
obliga que la solución cumpla con la dinámica del sistema
El problema de control óptimo
1. ¿Variables de decisión?
¿Por qué es complicado este problema?
\dim(\mathbf{u})=m
1. ¿Variables de decisión?
¿Por qué es complicado este problema?
\dim(\mathbf{u})=m
\dim\left(\mathbf{u}(t)\right)=
\dim\left( \begin{bmatrix} u_1(t) \\ u_2(t) \\ \vdots \\ u_m(t)\end{bmatrix} \right)
1. ¿Variables de decisión?
¿Por qué es complicado este problema?
\dim(\mathbf{u})=m
\dim\left(\mathbf{u}(t)\right)=
\dim\left( \begin{bmatrix} u_1(t) \\ u_2(t) \\ \vdots \\ u_m(t)\end{bmatrix} \right)
=\dim\left(u_1(t)\right)+\dim\left(u_2(t)\right)+\cdots+\dim\left(u_m(t)\right)
1. ¿Variables de decisión?
¿Por qué es complicado este problema?
\dim(\mathbf{u})=m
\dim\left(\mathbf{u}(t)\right)=
\dim\left( \begin{bmatrix} u_1(t) \\ u_2(t) \\ \vdots \\ u_m(t)\end{bmatrix} \right)
=\dim\left(u_1(t)\right)+\dim\left(u_2(t)\right)+\cdots+\dim\left(u_m(t)\right)
señales de tiempo continuo \(\Rightarrow \dim \to \infty\)
1. ¿Variables de decisión?
¿Por qué es complicado este problema?
\dim(\mathbf{u})=m
\dim\left(\mathbf{u}(t)\right)=
\dim\left( \begin{bmatrix} u_1(t) \\ u_2(t) \\ \vdots \\ u_m(t)\end{bmatrix} \right)
=\dim\left(u_1(t)\right)+\dim\left(u_2(t)\right)+\cdots+\dim\left(u_m(t)\right) \\
=m \times \infty = \infty
1. ¿Variables de decisión?
¿Por qué es complicado este problema?
\dim(\mathbf{u})=m
\dim\left(\mathbf{u}(t)\right)=
\dim\left( \begin{bmatrix} u_1(t) \\ u_2(t) \\ \vdots \\ u_m(t)\end{bmatrix} \right)
=\dim\left(u_1(t)\right)+\dim\left(u_2(t)\right)+\cdots+\dim\left(u_m(t)\right) \\
=m \times \infty = \infty
(!)
1. ¿Variables de decisión?
¿Por qué es complicado este problema?
2. Resticciones
\textrm{s.t.} \quad \dot{\mathbf{x}}=\mathbf{f}\left(\mathbf{x}, \mathbf{u}\right)
restricción de igualdad en forma de una ecuación diferencial vectorial no lineal
¿Por qué es complicado este problema?
2. Resticciones
¿Por qué es complicado este problema?
\textrm{s.t.} \quad \dot{\mathbf{x}}=\mathbf{f}\left(\mathbf{x}, \mathbf{u}\right)
restricción de igualdad en forma de una ecuación diferencial vectorial no lineal
(!)
-
analíticas
- cálculo de variaciones
- teoría de Hamilton-Jacobi-Bellman (dynamic programming)
- principio del máximo de Pontryagin
-
numéricas
- requieren transcripción a un programa no lineal
¿Qué herramientas existen para resolver?
-
analíticas
- cálculo de variaciones
- teoría de Hamilton-Jacobi-Bellman (dynamic programming)
- principio del máximo de Pontryagin
-
numéricas
- requieren transcripción a un programa no lineal
¿Qué herramientas existen para resolver?
\begin{aligned}
& \displaystyle\min_{\mathbf{u(t)}} \displaystyle\int_{t_0}^{t_f} \mathcal{L}\left(\mathbf{x}(\tau), \mathbf{u}(\tau) \right)d\tau + \varphi\left(\mathbf{x}_0,\mathbf{x}_f\right) \\
& \textrm{s.t.} \quad \dot{\mathbf{x}}=\mathbf{f}\left(\mathbf{x}, \mathbf{u}\right) \\
\end{aligned}
Transcripción directa
\begin{aligned}
& \displaystyle\min_{\mathbf{u(t)}} \displaystyle\int_{t_0}^{t_f} \mathcal{L}\left(\mathbf{x}(\tau), \mathbf{u}(\tau) \right)d\tau + \varphi\left(\mathbf{x}_0,\mathbf{x}_f\right) \\
& \textrm{s.t.} \quad \dot{\mathbf{x}}=\mathbf{f}\left(\mathbf{x}, \mathbf{u}\right) \\
\end{aligned}
\begin{aligned}
& \displaystyle\min_{\mathbf{z}} & f\left(\mathbf{z}\right) \qquad \\
& \text{s.t.} & \mathbf{g}_i\left(\mathbf{z}\right) \le\mathbf{0} \quad \forall i \\
& & \mathbf{h}_j\left(\mathbf{z}\right)=\mathbf{0} \quad \forall j
\end{aligned}
Transcripción directa
\begin{aligned}
& \displaystyle\min_{\mathbf{u(t)}} \displaystyle\int_{t_0}^{t_f} \mathcal{L}\left(\mathbf{x}(\tau), \mathbf{u}(\tau) \right)d\tau + \varphi\left(\mathbf{x}_0,\mathbf{x}_f\right) \\
& \textrm{s.t.} \quad \dot{\mathbf{x}}=\mathbf{f}\left(\mathbf{x}, \mathbf{u}\right) \\
\end{aligned}
\begin{aligned}
& \displaystyle\min_{\mathbf{z}} & f\left(\mathbf{z}\right) \qquad \\
& \text{s.t.} & \mathbf{g}_i\left(\mathbf{z}\right) \le\mathbf{0} \quad \forall i \\
& & \mathbf{h}_j\left(\mathbf{z}\right)=\mathbf{0} \quad \forall j
\end{aligned}
primero discretizamos y luego optimizamos
Transcripción directa
sencillos de plantear y resolver pero menos precisos
Transcripción directa
sencillos de plantear y resolver pero menos precisos
¿Transcripción indirecta?
presentan mejor precisión pero requieren de las técnicas analíticas para su planteamiento
Transcripción directa
sencillos de plantear y resolver pero menos precisos
¿Transcripción indirecta?
presentan mejor precisión pero requieren de las técnicas analíticas para su planteamiento
\(\Rightarrow\) NO los emplearemos
Transcripción directa
-
métodos de disparo (shooting)
- basados en simulación
- buenos para problemas sin (muchas) restricciones
-
métodos de colocación (collocation)
- basados en aproximaciones de la dinámica
- mejores para problemas con restricciones
Dos métodos
Shooting vs Collocation
-
métodos de disparo (shooting)
- basados en simulación
- buenos para problemas sin (muchas) restricciones
-
métodos de colocación (collocation)
- basados en aproximaciones de la dinámica
- mejores para problemas con restricciones
método de colocación directa
Dos métodos
IE3041 - Lecture 12 (2025)
By Miguel Enrique Zea Arenales
