Preferences, Indifference Curves, and Utility Functions
Christopher Makler
Stanford University Department of Economics
Econ 50: Lecture 2
Friday
Monday
Wednesday
Preferences & Utility
Marginal Rate of Substitution
Utility Function Examples
Budget Constraints
Utility Maximization subject to a Budget Constraint
Cases when
Calculus Doesn't Work
Demand Functions and Demand Curves
Midterm I
Decomposing a Price Change into Income & Substitution Effects
Demand Curve Shifters: Complements & Substitutes
Unit I: Consumer Theory
Welcome
Week 1
Week 2
Week 3
Week 4
Cost Minimization
Friday
Monday
Wednesday
Preferences & Utility
Marginal Rate of Substitution
Utility Function Examples
Unit I: Consumer Theory
Week 1
Week 2
Module 1: Modeling Consumer Preferences
Module 1: Modeling Consumer Preferences
Think about a choice you've had to make:
big or small, profound or trivial. How did you choose?
Introduce yourself to your neighbor,
and tell them about this choice.
Preferences: Ordinal Ranking of Options
Given a choice between option A and option B, an agent might have different preferences:
A \succ B
A \succeq B
A \sim B
A \preceq B
A \prec B
The agent strictly prefers A to B.
The agent strictly disprefers A to B.
The agent weakly prefers A to B.
The agent weakly disprefers A to B.
The agent is indifferent between A and B.
Sidebar: “Strictly" vs. “Weakly"
A \succ B
A \succeq B
The agent strictly prefers A to B.
The agent weakly prefers A to B.
2
3
4
5
6
x \ge 3
x \gt 3
Preference Axioms
Complete
Transitive
Any two options can be compared.
If \(A\) is preferred to \(B\), and \(B\) is preferred to \(C\),
then \(A\) is preferred to \(C\).
\text{For any options }A\text{ and }B\text{, either }A \succeq B \text{ or } B \succeq A
\text{If }A \succeq B \text{ and } B \succeq C\text{, then } A \succeq C
Together, these assumptions mean that we can rank
all possible choices in a coherent way.
For any choice, the choice space is the set of all options you're choosing between.
One such choice space
is the set of all bundles of commodity goods.
A=(4,3,6)
Example: “good 1” is apples, “good 2” is bananas, and
“good 3” is cherries
🍏🍏🍏🍏
🍌🍌🍌
🍒🍒🍒🍒🍒🍒
B=(3,8,2)
🍏🍏🍏
🍌🍌🍌🍌🍌🍌🍌🍌
🍒🍒
vectors
A=(4,3,6)
Example: “good 1” is apples, “good 2” is bananas, and
“good 3” is cherries
🍏🍏🍏🍏
🍌🍌🍌
🍒🍒🍒🍒🍒🍒
B=(3,8,2)
🍏🍏🍏
🍌🍌🍌🍌🍌🍌🍌🍌
🍒🍒
TWO NOTES
- Flows (e.g. apples per week) not stocks (apples)
- All quantities are infinitely divisible in this course.
Choices in general
Choices of commodity bundles
Choosing bundles of two goods
Special Case: Good 1 - Good 2 Space
x_1
x_2
Two "Goods" (e.g. apples and bananas)
A bundle is some quantity of each good
\text{Bundle }X = (x_1,x_2)
x_1 = \text{quantity of good 1 in bundle }X
x_2 = \text{quantity of good 2 in bundle }X
Can plot this in a graph with \(x_1\) on the horizontal axis and \(x_2\) on the vertical axis
A = (4,16)
B = (8,8)
A
B
4
8
12
16
20
4
8
12
16
20
Good 1 \((x_1)\)
Good 2 \((x_2)\)
Completeness axiom:
any two bundles can be compared.
Implication: given any bundle \(A\),
the choice space may be divided
into three regions:
preferred to A
dispreferred to A
indifferent to A
Indifference curves cannot cross!
A
The indifference curve through A connects all the bundles indifferent to A.
Indifference curve
through A
Special Case: Good 1 - Good 2 Space
How do we represent preferences mathematically?
How do I love thee? Let me count the ways.
I love thee to the depth and breadth and height
My soul can reach, when feeling out of sight
For the ends of Being and ideal Grace.
I love thee to the level of everyday’s
Most quiet need, by sun and candlelight.
I love thee freely, as men strive for Right;
I love thee purely, as they turn from Praise.
I love thee with the passion put to use
In my old griefs, and with my childhood’s faith.
I love thee with a love I seemed to lose
With my lost saints,—I love thee with the breath,
Smiles, tears, of all my life!—and, if God choose,
I shall but love thee better after death.
Elizabeth Barrett Browning
Sonnets from the Portugese 43
18th/19th Centuries: Utilitarianism
the greatest happiness of the greatest number
is the foundation of morals and legislation.
Jeremy Bentham
the utilitarian standard...
is not the agent's own greatest happiness,
but the greatest amount of happiness, altogether.
John Stuart Mill
Introduction to the Principles of Morals and Legislation (1789)
Utilitarianism (1861)
Preferences: Ordinal Ranking of Options
Given a choice between option A and option B, an agent might have different preferences:
A \succ B
A \succeq B
A \sim B
A \preceq B
A \prec B
The agent strictly prefers A to B.
The agent strictly disprefers A to B.
The agent weakly prefers A to B.
The agent weakly disprefers A to B.
The agent is indifferent between A and B.
Representing Preferences with a Utility Function
u(A) > u(B)
u(A) \ge u(B)
u(A) = u(B)
u(A) \le u(B)
u(A) < u(B)
u()
"A is strictly preferred to B"
Words
Preferences
Utility
A \succ B
A \succeq B
A \sim B
A \preceq B
A \prec B
"A is weakly preferred to B"
"A is indifferent to B"
"A is weakly dispreferred to B"
"A is strictly dispreferred to B"
Suppose the "utility function"
assigns a real number (in "utils")
to every possible consumption bundle
We get completeness because any two numbers can be compared,
and we get transitivity because that's a property of the operator ">"
u(A)
A=(4,3,6)
🍏🍏🍏🍏
🍌🍌🍌
🍒🍒🍒🍒🍒🍒
vector
😁😁😁😁
We need a mathematical function that maps vectors onto numbers.
number of "utils"
Multivariable Functions
x
f()
z = f(x,y)
y
z
[INDEPENDENT VARIABLES]
[DEPENDENT VARIABLE]
\text{Level set for }z=\{(x,y)|f(x,y)=z\}
An indifference curve is a set of all bundles between which a consumer is indifferent.
If a consumer is indifferent between two bundles (A ~ B), then \(u(A) = u(B)\)
Therefore, an indifference curve is a set of all consumption bundles which are assigned the same number of "utils" by the function \(u(x_1,x_2)\)
Likewise, set of bundles preferred to some bundle A is the a set of all consumption bundles which are assigned a greater number of "utils" by \(u(x_1,x_2)\)
Question: do we have to take "utils" seriously?
We can see if one bundle is preferred to another by comparing their utilities:
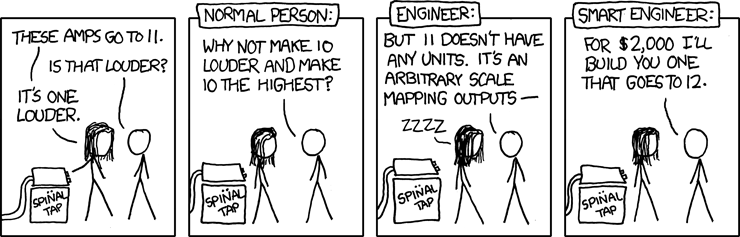
From XKCD:
Just like the "volume" on an amp, "utils" are an arbitrary scale...saying you're "11" happy from a bundle doesn't mean anything!
All we want to use utility functions for
is to describe preference orderings.
It doesn't matter that “utils" are nonsense.
As long as the utility function generates the correct indifference map,
it doesn't matter what the level of utility at each indifference curve is.
Transforming Utility Functions
Applying a positive monotonic transformation to a utility function doesn't affect
the way it ranks bundles.
u(x_1,x_2) = x_1^{1 \over 2}x_2^{1 \over 2}
\hat u(x_1,x_2) = 2x_1^{1 \over 2}x_2^{1 \over 2}
Example: \(\hat u(x_1,x_2) = 2u(x_1,x_2)\)
u(4,16) = 8
u(9,4) = 6
u(4, 16) = 2 \times 8 = 16
u(9,4) = 2 \times 6 = 12
pollev.com/chrismakler
The utility function \(u(x_1,x_2) = x_1x_2^2\) represents the same preferences as which of the following utility functions? You may select more than one answer.
Normalizing Utility Functions
One reason to transform a utility function is to normalize it.
This allows us to describe preferences using fewer parameters.
u(x_1,x_2) = ax_1 + bx_2
{1 \over a + b} u(x_1,x_2) = {a \over a + b}x_1 + {b \over a + b}x_2
\hat u(x_1,x_2) = \alpha x_1 + (1 - \alpha)x_2
[ multiply by \({1 \over a + b}\) ]
[ let \(\alpha = {a \over a + b}\) ]
= {a \over a + b}x_1 + \left [1 - {a \over a + b}\right]x_2
Summary
- Underlying the model of consumer choice is a model of preferences.
- We can use multivariate utility functions to represent preferences; indifference curves are level sets of utility functions.
- Preferences are real; "utils" are not. Any two utility functions which generate the same indifference curves represent the same preferences.
- Homework 1 (due next Tuesday) and the quiz for Friday's readings will be posted later this afternoon.
- Sections start today!! You'll be going over practical advice on how to draw a level set from a multivariate function, which is something a lot of people have a hard time with! (Plus, some math review.)
Econ 50 | Spring 25 | Lecture 02
By Chris Makler
Econ 50 | Spring 25 | Lecture 02
Preferences, Indifference Curves, and Utility Functions
