Nash Equilibrium
Christopher Makler
Stanford University Department of Economics
Econ 51: Lecture 9
pollev.com/chrismakler

Choose an integer between 0 and 100.
The student(s) with the guess closest to 70% of the class average as of 9:35am gets 3 extra homework points.
-
Iterated deletion of strictly dominated strategies
-
Second strategic tension: strategic uncertainty
-
Nash equilibrium
-
in pure strategies
-
in mixed strategies
-
-
Application to Cournot duopoly
Today's Agenda
Iterated Deletion of Strictly Dominated Strategies
Iterated Deletion of Dominated Strategies
(a.k.a. “Iterated Dominance")
- Suppose it is common knowledge that all players are rational,
so they will never choose a strictly dominated strategy. -
We can remove from the game any strictly dominated strategies
(by either a pure or mixed strategy). -
Removal may create new dominated strategies, remove them too…
-
The set of strategies that’s left at the end of that process
is the set of rationalizable strategies.-
turns out to be independent of the order
in which dominated strategies are removed
-
Which strategy or strategies is strictly dominated for a player?
1
2
1
2
,
4
3
,
1
4
,
1
1
,
Top
Middle
Left
Center
Bottom
Right
3
0
,
2
1
,
3
2
,
8
0
,
8
0
,
Center strictly dominates Right.
If we know that player 2 will never play Right, is any strategy now dominated for player 1?
Bottom strictly dominates Top.
And with that off the board...
Bottom strictly dominates Middle.
Can we eliminate anything else?
Center strictly dominates Left.
Cournot Duopoly
What is firm 2's best response function?
2
P
"Firm 2's Residual Demand Curve"
Firm 2's "best response function"
Firm 2's "best response function"
2
4
6
8
10
12
2
4
6
8
10
12
Firm 2's "best response function"
Firm 1's "best response function"
2
4
6
8
10
12
2
4
6
8
10
12
Applying iterated deletion:
if everyone knows everything about this model (and everyone knows that everyone knows everything about this model), what do each of the firms know about the other firm's beliefs?
Each firm knows the other
will never produce more than 6.
Because \(6 - {1 \over 2}6 = 3\),
this means each firm knows the other
will never produce less than 3.
Because \(6 - {1 \over 2}3 = 4.5\),
this means each firm knows the other
will never produce more than 4.5.
The only set of quantities that survives this is (4,4).
2
4
6
8
10
12
2
4
6
8
10
12
Keynesian Beauty Contest (70% Game)
Everyone chooses an integer between 0 and 100.
The closest person to 70% of the average guess wins.
0
100
70
DOMINATED BY 70
Keynesian Beauty Contest (70% Game)
Everyone chooses an integer between 0 and 100.
The closest person to 70% of the average guess wins.
0
100
70
49
DOMINATED BY 70
DOMINATED
BY 49
Keynesian Beauty Contest (70% Game)
Everyone chooses an integer between 0 and 100.
The closest person to 70% of the average guess wins.
0
100
70
49
34.3
DOMINATED
BY 49
DOM'D
BY 34.3
DOMINATED BY 70
Keynesian Beauty Contest (70% Game)
Everyone chooses an integer between 0 and 100.
The closest person to 70% of the average guess wins.
0
100
70
49
34.3
24
DOM'D
BY 34.3
DOM'D
BY 24
DOMINATED
BY 49
DOMINATED BY 70
Keynesian Beauty Contest (70% Game)
Everyone chooses an integer between 0 and 100.
The closest person to 70% of the average guess wins.
0
100
Iterated deletion of strictly dominated strategies tells us the only rationalizable strategy is 0.
Why didn't everyone choose 0?
Second Strategic Tension:
Strategic Uncertainty
Stag Hunt Game
-
Proposed by philosopher Jean-Jacques Rousseau in his Discourse on Inequality (1755)
-
Two hunters independently choose whether to hunt a stag or a hare.
-
A player who chooses to hunt a hare always gets a payoff of 4 regardless of the other player's choice.
-
A stag hunt only succeeds if both players do it.
In that case, the players divide the stag, giving a payoff of 5 to each. -
But if only one player hunts a stag, he fails and gets a payoff of zero.
1
2
Stag
Hare
Stag
Hare
3
3
,
2
0
,
2
2
,
0
2
,
Homework Hunt Game
pollev.com/chrismakler

If you choose A, you get \(3p\) homework points, where \(p\) is the fraction of the class choosing A.
If you choose B, you get 2 points no matter what.
1
2
A
B
A
B
3
3
,
2
0
,
2
2
,
0
2
,
If fraction \(p\) of the class choose A...
3p
2
Best Response: From Last Time
In plain English: given my beliefs about what the other player(s) are doing, a strategy is my "best response"
if there is no other strategy available to me
that would give me a higher payoff.
Best Response under Strategic Certainty
In plain English: given the strategies chosen by the other player(s),
a strategy is my "best response"
if there is no other strategy available to me
that would give me a higher payoff.
Possible rationales for strategic certainty:
- People play this game all the time, and reasonably expect
the other player to play according to the equilibrium. - The players have agreed on a strategy before the game is played;
as long as no one has an incentive to deviate, it's OK. - An outside mediator (society, the law) recommends a strategy profile
Stag Hunt Game with Strategic Certainty
1
2
Stag
Hare
Stag
Hare
3
3
,
2
0
,
2
2
,
0
2
,
Equilibrium
Definition: Best Response (Nash) Equilibrium
In plain English: in a Nash Equilibrium, every player is playing a best response to the strategies played by the other players.
In other words: there is no profitable unilateral deviation
given the other players' equilibrium strategies.
Nash Equilibrium
with Pure Strategies
How class participation will count toward your grade
- I will use PollEv to keep track of who has attended each lecture
- If you do badly on an exam question, I will look to see if you missed class on the day related to that question.
- If you were in class or had an excused absence*, I will add a few points back. If you just skipped class that day, I will have less sympathy.
- So: you can still get 100% if you never come to class and ace all the exams; but if you do badly on an exam, showing that you were in class can help mitigate the damage.
- *You may not have too many excused absences. (You can't just call in sick for the rest of the term.)
1
2
1
2
,
4
3
,
1
4
,
1
1
,
T
M
L
C
B
R
3
0
,
2
1
,
3
2
,
8
0
,
8
0
,
Nash equilibrium occurs when every player is choosing strategy which is a
best response to the strategies chosen by the other player(s)
1
2
1
2
,
4
3
,
1
4
,
1
1
,
T
M
L
C
B
R
3
0
,
2
1
,
3
2
,
8
0
,
8
0
,
Nash equilibrium occurs when every player is choosing strategy which is a
best response to the strategies chosen by the other player(s)
Because B is a best response to C,
and C is a Best response to B,
the strategy profile (B,C)
is a Nash Equilibrium.
IMPORTANT: THE NASH EQUILIBRIUM IS THE STRATEGY PROFILE (B,C), NOT THE PAYOFFS (2,1)!

Multiple Equilibria

Coordination Game
1
2
Drive on Left
Drive on Right
Drive on Left
Drive on Right
1
1
,
0
0
,
1
1
,
0
0
,
pollev.com/chrismakler

Pareto Coordination
1
2
A
B
A
B
2
2
,
0
0
,
1
1
,
0
0
,
pollev.com/chrismakler

Cournot Equilibrium
Cournot Best Response Functions
Firm 1's
best response function
Firm 2's
best response function
Nash equilibrium (a.k.a. Cournot equilibrium) is a pair of quantities \((q_1,q_2)\) such that
Profits in Cournot Equilibrium
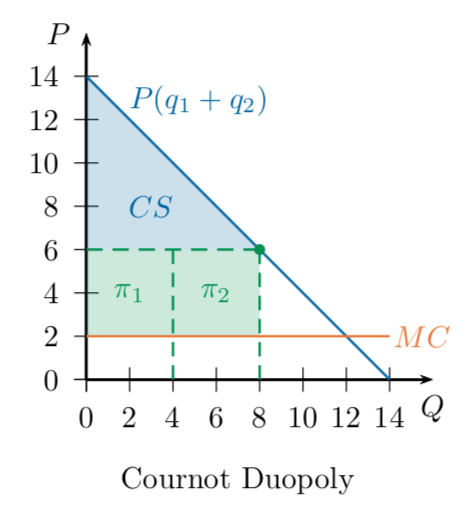
Each firm is producing 4 units, so the market price is \(14 - 4 - 4 = 6\).
Each unit costs $2, so each firm is making
$4 of profit on 4 units = $16.
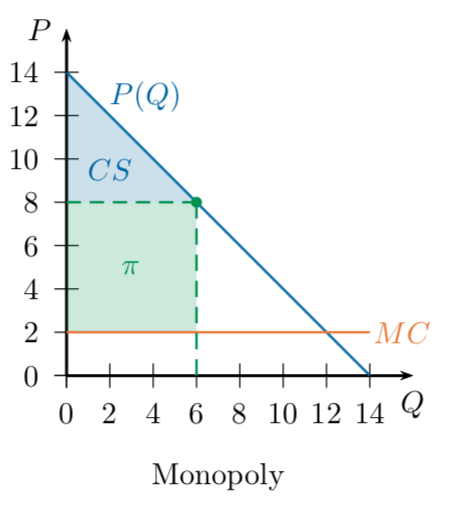
Remember our monopoly: it produced 6 units,
sold them at a price of 8, and earned a total profit of 36.
If each of these two firms produced 3 units, they could earn 18...
so why don't they?
Next Thursday, we'll look at collusion between firms.
Nash Equilibrium
with Mixed Strategies
Matching Pennies I: Coordination Game
1
2
Heads
Tails
Heads
Tails
1
1
,
-1
-1
,
1
1
,
-1
-1
,
Each player chooses Heads or Tails.
If they choose the same thing,
they both "win" (get a payoff of 1).
If they choose differently,
they both "lose" (get a payoff of -1).
Circle best responses.
What are the Nash equilibria of this game?
Matching Pennies II: Zero-Sum Game
1
2
Heads
Tails
Heads
Tails
1
-1
,
-1
1
,
1
-1
,
-1
1
,
Each player chooses Heads or Tails.
If they choose the same thing,
player 1 "wins" (gets a payoff of 1)
and player 2 "loses" (gets a payoff of -1).
If they choose differently,
they player 1 "loses" (gets a payoff of -1)
and player 1 "wins" (gets a payoff of 1).
Circle best responses.
What are the Nash equilibria of this game?
Best Response
If two or more pure strategies are best responses given what the other player is doing, then any mixed strategy which puts probability on those strategies (and no others) is also a best response.
2
\({1 \over 6}\)
\({1 \over 3}\)
\({1 \over 2}\)
\(0\)
Player 2's strategy
\({1 \over 6} \times 6 + {1 \over 3} \times 3 + {1 \over 2} \times 2 + 0 \times 7\)
\(=3\)
\({1 \over 6} \times 12 + {1 \over 3} \times 6 + {1 \over 2} \times 0 + 0 \times 5\)
\(=4\)
\({1 \over 6} \times 6 + {1 \over 3} \times 0 + {1 \over 2} \times 6 + 0 \times 11\)
\(=4\)
Player 1's expected payoffs from each of their strategies
\(X\)
\(A\)
1
\(B\)
\(C\)
\(D\)
\(Y\)
\(Z\)
6
6
,
3
6
,
2
8
,
7
0
,
12
6
,
6
3
,
0
2
,
5
0
,
6
0
,
0
9
,
6
8
,
11
4
,
If player 2 is choosing this strategy, player 1's best response is to play either Y or Z.
Therefore, player 1 could also choose to play any mixed strategy \((0, p, 1-p)\).
When is a mixed strategy a best response?
1
2
Heads
Tails
Heads
Tails
1
-1
,
-1
1
,
1
-1
,
-1
1
,
Let's return to our zero-sum game.
\((p)\)
\((1-p)\)
What is player 1's expected payoff from Heads?
Suppose player 2 is playing a mixed strategy: Heads with probability \(p\),
and tails with probability \(1-p\).
What is player 1's expected payoff from Tails?
For what value of \(p\) would player 1 be willing to mix?
When is a mixed strategy a best response?
1
2
Heads
Tails
Heads
Tails
1
-1
,
-1
1
,
1
-1
,
-1
1
,
\((p)\)
\((1-p)\)
For what value of \(p\) would player 1 be willing to mix?
Now suppose player 1 does mix, and plays Heads with probability \(q\) and Tails with probability \(1 - q\).
\((q)\)
\((1-q)\)
For what value of \(q\) would player 2 be willing to mix?
Equilibrium in Mixed Strategies
A mixed strategy profile is a Nash equilibrium if,
given all players' strategies, each player is mixing among strategies which are their best responses
(i.e. between which they are indifferent)
Important: nobody is trying to make the other player(s) indifferent; it's just that in equilibrium they are indifferent.
Next Week
- How does introducing time affect the kinds of outcomes we can observe in equilibrium?
Econ 51 | 9 | Nash Equilibrium
By Chris Makler
Econ 51 | 9 | Nash Equilibrium
Nash equilibrium in pure and mixed strategies
- 953



